Number (patterns): Honeycomb
The Problem
Mr Buzalot was a beekeeper. He was also a keen mathematician. Fascinated by the honeycombs that were inside his beehives and their repeating hexagonal forms, he decided to copy the pattern using lollipop sticks.
Mr Buzalot was curious to find answers to the following questions:
- What is the highest number of lollipop sticks that would be needed to make 6 hexagons with one or more shared sides?
- What is the lowest number of lollipop sticks that would be needed to make 6 hexagons with one or more shared sides?
Can you help Mr Buzalot find answers to these questions?
How do you know if you have found the highest/lowest number of lollipop sticks?

Did you know?
Collecting honey from wild bee colonies is one of the most ancient human activities. The practice of beekeeping to harvest honey dates back at least 9,000 years!
Visualising the Problem and Getting Started
Before attempting to solve the main problem, see if you can construct a single hexagon from 6 lollipop sticks. Once you have done this, see if you can construct 2 hexagons from fewer than 12 sticks. You will notice that the 2 hexagons have a shared side.
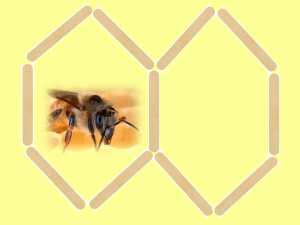
Iskra said, “You will always need 11 lollipop sticks when making 2 hexagons with one or more shared sides, even when the hexagons are in different places.” Do you agree with Iskra?

 Sign in
Sign in

